路径总和

112. 路径总和

给你二叉树的根节点 root 和一个表示目标和的整数 targetSum 。判断该树中是否存在 根节点到叶子节点 的路径，这条路径上所有节点值相加等于目标和 targetSum 。如果存在，返回 true ；否则，返回 false 。

叶子节点 是指没有子节点的节点。

示例 1：

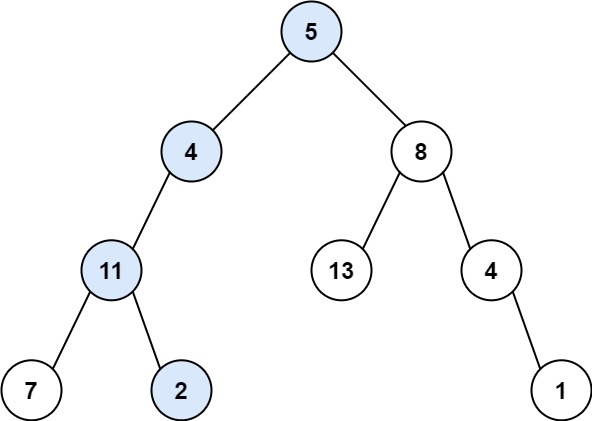
输入：root = [5,4,8,11,null,13,4,7,2,null,null,null,1], targetSum = 22
输出：true
解释：等于目标和的根节点到叶节点路径如上图所示。

示例 2：

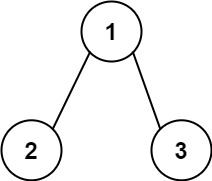
输入：root = [1,2,3], targetSum = 5
输出：false
解释：树中存在两条根节点到叶子节点的路径：
(1 --> 2): 和为 3
(1 --> 3): 和为 4
不存在 sum = 5 的根节点到叶子节点的路径。

示例 3：

输入：root = [], targetSum = 0
输出：false
解释：由于树是空的，所以不存在根节点到叶子节点的路径。

提示：

• 树中节点的数目在范围 [0, 5000] 内
• -1000 <= Node.val <= 1000
• -1000 <= targetSum <= 1000

迭代（后序遍历）

class Solution {
    
    public boolean hasPathSum(TreeNode root, int targetSum) {
         Stack<TreeNode> st = new Stack<>();
         TreeNode pre = null;
         while(root != null || !st.isEmpty()){
             while(root != null){
                 st.push(root);
                 root = root.left;
             }
             root = st.peek();
             if(root.right == null || pre == root.right){//右节点未被访问或为空，访问该节点
                if(root.left == null && root.right ==null){//遍历到叶子节点
                    int pathSum = 0; //记录当前路径的总和
                    for(TreeNode node : st){
                        pathSum += node.val;
                    }
                    if(pathSum == targetSum){
                        return true;
                    }
                }
                root = st.pop(); //访问节点出栈
                pre = root;
                root = null;
             }else{
                 root = root.right;
             }
         }
         return false;
    }
    
}

回溯

class Solution {
    int pathSum = 0; //记录当前路径的总和
    public boolean hasPathSum(TreeNode root, int targetSum) {
         return has_sum(root,targetSum);
    }
    
    //只需要找一条满足的路径，故返回boolean
    public boolean has_sum(TreeNode root,int targetSum){
        if(root==null) return false;
        pathSum += root.val;
        if(root.left==null&&root.right==null){
            if(pathSum==targetSum){
               return true;
            }
        }
        
        if(root.left!=null){
            if(has_sum(root.left,targetSum)) return true;//找到一条路径返回true，没有找到继续遍历
            pathSum -= root.left.val;
        }
        if(root.right!=null){
            if(has_sum(root.right,targetSum)) return true;
            pathSum -= root.right.val; 
        }
        return false;
        
    }
}